1 Answer

Marcos Marin · Answer 1 · 2023-05-13T10:44:54+0000

Answer:

AB = 9.2cm (to the nearest tenth).

Step-by-step explanation:

Chords CE and GF intersect at point D.

First, we apply the theorem of intersecting chords:

$\begin{gathered} CD* DE=DG* DF \\ CD*2=4*3 \\ CD=(12)/(2) \\ CD=6\operatorname{cm} \end{gathered}$

Next, AB is a tangent while line BE is a secant.

AB and BE intersect at B.

Using the theorem of intersecting tangent and secant:

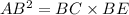
First, find the length of BE.

$\begin{gathered} BE=BC+CD+DE \\ =6+6+2 \\ BE=14\operatorname{cm} \end{gathered}$

Substitute BE=14cm and BC=6 cm into the formula:

$\begin{gathered} AB^2=BC* BE \\ AB^2=6*14 \\ AB^2=84 \\ AB=\sqrt[]{84} \\ AB=9.2\text{ cm (to the nearest tenth)} \end{gathered}$

The length of AB is 9.2cm (to the nearest tenth).

Your comment on this question: