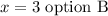Question

which value of x is a solution to this equation?2x² - 2x - 12 = 0A) x = -1.5B) x = 3 C) x = -3D) x = 2

Answer 1

Step 1

Given;

`\begin{gathered} 2x^2-2x-12=0 \\ \end{gathered}`

Required; To find the value of x

Step 2

Factorize the equation.

`Divide\text{ all terms by 2}`
`\begin{gathered} (2x^2)/(2)-(2x)/(2)-(12)/(2)=(0)/(6) \\ x^2-x-6=0 \end{gathered}`

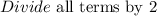
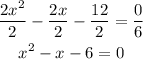
Factors required to factorize the problem are -3x and 2x. We will now replace -x with these factors. That is -x=(-3x+2x)

`\begin{gathered} x^2-3x+2x-6=0 \\ (x^2-3x)(+2x-6)=0 \end{gathered}`
`\begin{gathered} Simplify \\ x((x^2)/(x)-(3x)/(x))+2((2x)/(2)-(6)/(2))=0 \\ x(x-3)+2(x-3)=0 \\ (x+2)(x-3)=0 \\ x=-2\text{ or 3} \end{gathered}`

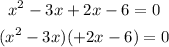
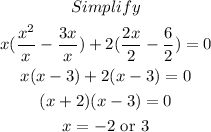
Hence, based on the options, the value of which is a solution to the equation is;

`x=3`

Answer;

`x=3\text{ option B}`