Question

Reconsider the collision problem above. This time one is headed north with a mass of1,375 kg at a velocity of 21 m/s. The other is headed south with a mass of 1,124 kg at avelocity 14 m/s. They collide and stick together.What is the IMPULSE for the car headed south?

Answer 1

The initial momentum of the system is,

`p_i=m_1u_1+m_2u_2`

The final momentum of the system is,

`p_f=(m_1+m_2)v`

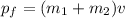
According to conservation of momentum,

`p_i=p_f`

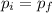
Plug in the known values,

`\begin{gathered} m_1u_1+m_2u_2=(m_1+m_2)v \\ v=(m_1u_1+m_2u_2)/(m_1+m_2) \end{gathered}`

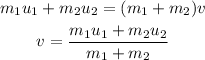
Substitute the known values,

`\begin{gathered} v=\frac{(1375\text{ kg)(21 m/s)+}(1124\text{ kg)(-14 m/s)}}{(1375\text{ kg+1124 kg)}} \\ =\frac{28875\text{ kgm/s-}15736\text{ kgm/s}}{2499\text{ kg}} \\ =\frac{13139\text{ kgm/s}}{2499\text{ kg}} \\ =5.26\text{ m/s} \end{gathered}`

The impulse of the car can be given as,

`J=m_2(u_2-v)`

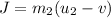
Plug in the known values,

`\begin{gathered} J=(1124\text{ kg)(}14\text{ m/s-5.26 m/s)} \\ =(1124\text{ kg)(}8.74\text{ m/s)} \\ =9823.76\text{ kgm/s} \end{gathered}`

Therefore, the impulse of the car headed south is 9823.76 kgm/s.