Question

A total of $7000 is invested: part at 8% and the remainder at 12%. How much is invested at each rate if the annual interest is $580?

Answer 1

We invested $7000 invested in two accounts, we don't know how much exactly is in each account, therefore we will assign variables to these amounts. The amount invested in the "8%" account is "x", while the other one is "y". The sum of these two numbers must be equal to $7000, therefore:

`x+y=7000`

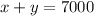
The sum of interest of each account should be equal to 580, therefore:

`0.08\cdot x+0.12\cdot y=580`

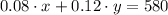
We need to solve the system in order to determine the values.

`\begin{gathered} \begin{cases}x+y=7000 \\ 0.08x+0.12y=580\end{cases} \\ \begin{cases}-0.12x-0.12y=-840 \\ 0.08x+0.12y=580\end{cases} \\ -0.04x=-260 \\ x=(-260)/(-0.04)=6500 \\ \end{gathered}`

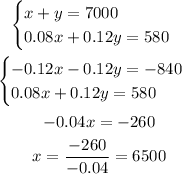
Now we can replace the value of "x" in the first equation to determine the value of "y".

`\begin{gathered} 6500+y=7000 \\ y=7000-6500 \\ y=500 \end{gathered}`

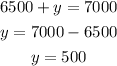
The values are 6500 in the 8% account and 500 in the 12% account.