Question

triangle PQR has vertices P(0,0), Q(8,-4) and R(-6,4). Find the vertices of P'Q'R' after each translationand on problem C there is a R' i just couldn't scroll all the way down

Answer 1

The translation of 6 units right increase the x-coordinate by 6 and 5 units up increase the y -coordinate by 5 units.

So coordinates of triangle P'Q'R' after translation is,

`P^(\prime)(0+6,0+5)\rightarrow P^(\prime)(6,5)`
`Q^(\prime)(8+6,-4+5)\rightarrow Q^(\prime)(14,1)`
`R^(\prime)(-6+6,4+5)\rightarrow R^(\prime)(0,9)`

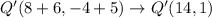
So new coordinates are,

P'(6,5)

Q'(14,1)

R'(0,9)

PART B.

The translation of 8 units left means that x-coordinate decrease by 8 units and 2 unit down means that y-coordinate decrease by 2 units.

Determine the coordinates of vertices of triangle after trnaslation.

`P^(\prime)(0-8,0-2)\rightarrow P^(\prime)(-8,-2)`
`Q^(\prime)(8-8,-4-2)\rightarrow Q^(\prime)(0,-6)`
`R^(\prime)(-6-8,4-2)\rightarrow R^(\prime)(-14,2)`

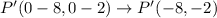
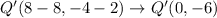
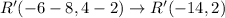
So coordinates of triangle P'Q'R' is,

P'(-8,-2)

Q'(0,-6)

R'(-14,2)

Part C:

The translation of 3 units right means x-coordinate increase by 3 and 4 units down means that y-coordinate decrease by 4.

Determine the coordinate of triangle after translation.

`P^(\prime)(0+3,0-4)\rightarrow P^(\prime)(3,-4)`
`Q^(\prime)(8+3,-4-4)\rightarrow Q^(\prime)(11,-8)`
`R^(\prime)(-6+3,4-4)\rightarrow R^(\prime)(-3,0)`

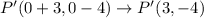
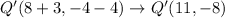
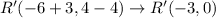
So coordinates of triangle P'Q'R' is,

P'(3,-4)

Q'(11,-8)

R'(-3,0)