Question

HELP PLEASE

How many solutions exist for the system of equations below?

3x+y=18
3x+y=16

none
one
two
infinite

Answer 1

The answer is none, as the same equation is equal to different numerical values

Answer 2

The correct option is 1.

Explanation:

The system of equation is

`3x+y=18`

`3x+y=16`

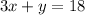
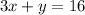
A system of equation has two equation
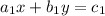`a_1x+b_1y=c_1` and
`a_2x+b_2y=c_2`.

1. If
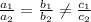`(a_1)/(a_2)=(b_1)/(b_2)\\eq (c_1)/(c_2)`, then system of equations has no solution.

2. If
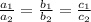`(a_1)/(a_2)=(b_1)/(b_2)=(c_1)/(c_2)`, then system of equations has infinite solution.

3. If
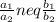`(a_1)/(a_2)neq (b_1)/(b_2)`, then system of equation has one solution.

In the given system of equations,

`a_1=3,b_1=1,c_1=8, a_2=3,b_2=1, c_2=16`

`(a_1)/(a_2)=(3)/(3)=1`

`(b_1)/(b_2)=(1)/(1)=1`

`(c_1)/(c_2)=(18)/(16)=(9)/(8)`

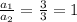
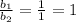
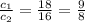
Since
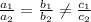`(a_1)/(a_2)=(b_1)/(b_2)\\eq (c_1)/(c_2)`, therefore the system of equation has no solution.

The correct option is 1.