Question 1: Is the investment increasing or decreasing?
Answer: Decreasing
Reason: Checking the factor (0.96)^t the factor became lesser the higher the value of t.
Example: (0.96)^1 = 0.96, (0.96)^2 = 0.9216, (0.96)^3 = 0.884736
Question 2: What was the initial investment?
Answer: 12,000
Question 3: How much will the investment be worth in 10 years?
Solution:
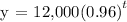
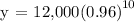
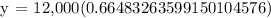
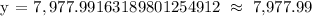
Answer: The investment will be worth 7,977.99 in 10 years.
Question 4: Find the annual sales in 7 years.
Equation:

Solution:

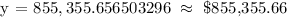
Answer: After 7 years, considering the annual rate increase of 4%, the annual sales will be $855,355.66