Question

Which of the following represents the distance between the y-intercept and x-intercept of the line whose equation is y=3x+12?(1) √120(2) √160(3) √200(4) √244

Answer 1

By definition, when the line cuts the x-axis, the value of "y" is:

`y=0`

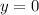
Therefore, to find the x-intercept of the line given in the exercise, you must substitute that value of "y" into the equation and solve for "x":

`\begin{gathered} 0=3x+12 \\ -12=3x \\ (-12)/(3)=x \\ x=-4 \end{gathered}`

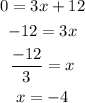
The equation of a line in Slope-Intercept form is:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept.

Kwnowing that the line is:

`y=3x+12`

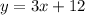
You can identify that the y-intercept is:

`b=12`

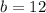
So, you have these points:

`(-4,0);(0,12)`

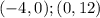
The formula used to find the distance between two points, is:

`d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

In this case:

`\begin{gathered} x_2=0 \\ x_1=-4 \\ y_2=12 \\ y_1=0 \end{gathered}`

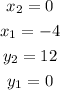
Substituting values and evaluating, you get that the distance between the y-intercept and x-intercept of the line, is:

`d=\sqrt[]{(0-(-4))^2+(12-0)^2}=\sqrt[]{16+144}=\sqrt[]{160}`

The answer is the option (2).