Question

Find the sum of {-7,-9,-11,-13,.....,-63}.

Answer 1

so... if you notice, it goes from -7, to -9 to -11 to -13

is going down by -2, so, if you "add" -2 to the current term, you get the next one, -7-2 = -9 and so on

thus, is an arithmetic sequence, and our "common difference" is -2

now, our first term is -7, our last one is -63, what's the ordinal value of the last one? is it the 5th? the 15th or what?

well
`\bf n^(th)\textit{ term of an arithmetic sequence}\\\\ a_n=a_1+(n-1)d\qquad \begin{cases} a_n=n^(th)\ term\\ a_1=\textit{first term}\\ d=\textit{common difference}\\ ----------\\ a_1=-7\\ d=-2\\ a_n=-63 \end{cases} \\\\\\ -63=-7+(n-1)(-2)\implies -63=-7-2n+2 \\\\\\ -63=-5-2n\implies -58=-2n\implies \cfrac{-58}{-2}=n \\\\\\ \boxed{29=n}`

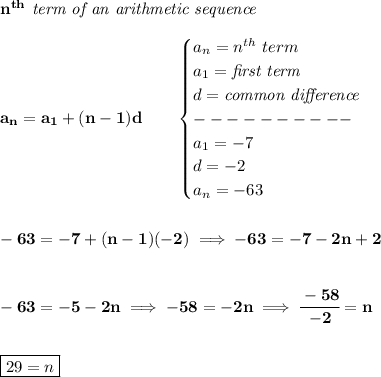
so.. .now we know, the -63 value, the last one, is really the 29th term

alrite, now, let's find their sum
`\bf \textit{sum of a finite arithmetic sequence}\\\\ S_n=\cfrac{n}{2}(a_1+a_n) \\\\\\ S_(29)=\cfrac{29}{2}[-7+(-63)]`