Question

Maria shoots a basketball at an angle of 40 from the horizontal. It leaves her hands 7 feet from the ground with a velocity of 20 ft/s.Step 1 of 2: Construct a set of parametric equations describing the shot. Round all final values to the nearest tenth.AnswerX=y =Previous

Answer 1

A projectile motion is projected at an angle of 40°, at a height of 7 feet, and at an initial velocity of 20 ft/s.

It is required to find the parametric equations that represent the motion.

Recall that the Parametric Equations that represent a projectile motion with angle θ, initial velocity v_0, and height h is given as:

`\begin{gathered} x=(v_0\cos\theta)t \\ y=-(1)/(2)gt^2+(v_0\sin\theta)t+h \end{gathered}`

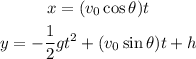
Where g=32 ft/s² is the acceleration due to gravity.

Substitute the constant g, the initial velocity, the height, and the angle of projection.

`\begin{gathered} x=(20\cos40^(\circ))t \\ y=-(1)/(2)(32)t^2+(20\sin40^(\circ))t+7 \end{gathered}`

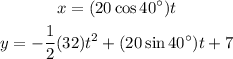
Simplify the equations and round final values to the nearest tenth:

`\begin{gathered} x=15.3t \\ y=-16t^2+12.9t+7 \end{gathered}`

Hence, the required parametric equations.