Question

Question 4 of 10Select the two binomials that are factors of this trinomial.x2 + 3x - 28O A. X-4B. X-7O C. X+7O D. X+ 4

Answer 1

Step-by-step explanation: Imagine you have the following generic trinomial

`ax^2+bx+c`

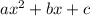
to find two binomials "x+n" and "x+m" for the trinomial above we just need to find the values for "n" and "m". We know that

`\begin{gathered} b=n+m \\ \text{and} \\ c=n\cdot m \end{gathered}`

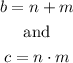
So finally we just need to find two numbers that the sum gives us the value of "b" and the product gives us the value of "c".

Step 1: Identify the variables

Once b = 3 and c = -28 we just need to find two numbers that the sum is equal to 3 and the product is equal to -28

Step 2: Now we can see that

`\begin{gathered} -4+7=3 \\ \text{and} \\ -4\cdot7=-28 \end{gathered}`

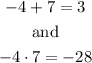
As we can see above the two numbers are -4 and +7.

Final answer: So the two binomials are

A. x-4 and C. x+7