Question

Select the correct answer.What is the solution to this equation?loge + + log 3 = log (+ + 1)OA1 =IO HICOB.1 =O C.सेNICOOD. r = 1

Answer 1

Given the equation:

`\log _6x+\log _63=\log _6(x+1)`

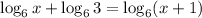
Let's solve for x.

To find the solution, take the following steps:

Step 1:

Apply the product property of logarithm to the left side of the equation:

`\begin{gathered} \log _6(x\ast3)=\log _6(x+1) \\ \\ \log _6(3x)=\log _6(x+1) \end{gathered}`

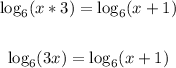
Step 2:

Eliminate the log on both sides

`\begin{gathered} 3x=(x+1) \\ \\ 3x=x+1 \end{gathered}`

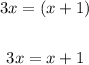
Step 3:

Subtract x from both sides

`\begin{gathered} 3x-x=x-x+1 \\ \\ 2x=1 \end{gathered}`

Step 4:

Divide both sides by 2

`\begin{gathered} (2x)/(2)=(1)/(2) \\ \\ x=(1)/(2) \end{gathered}`

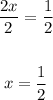
Therefore, the solution to the given equation is:

`x=(1)/(2)`

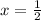
ANSWER:

`\text{ B. x = }(1)/(2)`