Question

Plot five points on the graph of the function:one point with x=0, two points with negative x-values and two points with positive x-values.

Answer 1

Given

`y=-(5)/(8)x^3`

First calculate the ordered pairs for each point, to do so, select the values of x, replace them in the equation of the function, and then calculate the corresponding y-values

I'll calculate the values for x=-2, x=-1, x=0, x=1, x=2

For x=-2

`\begin{gathered} y=-(5)/(8)(-2)^3 \\ y=(-(5)/(8))\cdot(-8) \\ y=5 \end{gathered}`

The first ordered pair is (-2,5)

For x=-1

`\begin{gathered} y=-(5)/(8)(-1)^3 \\ y=(-(5)/(8))(-1) \\ y=(5)/(8) \end{gathered}`

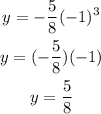
The second ordered pair is (-1,5/8)

For x=0

`\begin{gathered} y=-(5)/(8)\cdot0^3 \\ y=0 \end{gathered}`

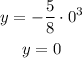
The third ordered pair is (0,0)

For x=1

`\begin{gathered} y=-(5)/(8)(1^3) \\ y=-(5)/(8) \end{gathered}`

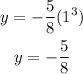
The fourth ordered pair is (1,-5/8)

For x=2

`\begin{gathered} y=-(5)/(8)(2^3) \\ y=-(5)/(8)\cdot8 \\ y=-5 \end{gathered}`

The fifth ordered pair is (2,-5)

Once you have determined all ordered pairs you can plot the five points.