Question

I need help solving check point 4. Please explain writing all the steps out.

Answer 1

First, find the LCM of the denominators (x-2) and 3. It is 3(x-2).

Multiply both sides of the equation by the LCM to cancel out all the denominators. Then, solve for x.

`\begin{gathered} (x)/(x-2)=(2)/(x-2)-(2)/(3) \\ \Rightarrow3(x-2)\mleft((x)/(x-2)\mright)=3(x-2)\mleft((2)/(x-2)-(2)/(3)\mright) \end{gathered}`

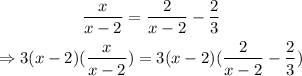
Expand the parenthesis on the right member of the equation:

`\Rightarrow3(x-2)\mleft((x)/(x-2)\mright)=3(x-2)\mleft((2)/(x-2)\mright)-3(x-2)\mleft((2)/(3)\mright)`

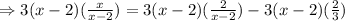
Cancel out the factors that appear on the denominator in each term:

`\Rightarrow3(x)=3(2)-(x-2)(2)`

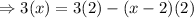
Expand the parenthesis on the right member of the equation:

`\Rightarrow3(x)=3(2)-(x)(2)+(2)(2)`

Expand all the parentheses and solve for x:

`\begin{gathered} \Rightarrow3x=6-2x+4 \\ \Rightarrow3x+2x=6+4 \\ \Rightarrow5x=6+4 \\ \Rightarrow5x=10 \\ \Rightarrow x=(10)/(5) \\ \therefore x=2 \end{gathered}`

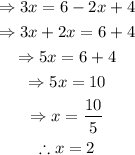
Notice that the expression x-2 appears in the denominator. Nevertheless, since the value of x that would make the equation true is 2, then the expression x-2 is equal to 0. On the other hand, the denominator cannot be equal to 0 because division over 0 is not defined.

Therefore, this equation has no solution (the solution set is the empty set).