Answer:
Explanation:
The given data set is:
27, 4, 54, 78, 27, 48, 79, 64, 5, 6, 41, 71
Firstly, arrange the given data set in ascending order, we get
4, 5, 6, 27, 27, 41, 48, 54, 64, 71, 78, 79
Now, the first half of the given data set is 4, 5, 6, 27, 27, 41 , thus the middle value is Lower quartile that is:
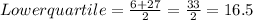
And, the second half of the given data set is 48, 54, 64, 71, 78, 79, thusthe middle value is the upper quartile that is:

Now, Interquartile range is given as:

=
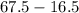
=
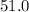
Thus, the Interquartile range is 51.