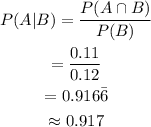Question

the probability that a randomly-selected IRSC student is an athlete is 0.16 the probability that a randomly-selected RSE student is a biology major is 0.12 the probability that a randomly-selected RS student is biology major in and out 30 is 0.11 calculate the following probability round the solution to the 3rd decimal place if necessary

Answer 1

The formula for the conditional probability is as follows:

`P(A|B)=(P(A\cap B))/(P(B))`

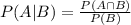
where P(A|B) is the probability of A given B, P(A∩B) is the probability of A and B, and P(B) is the probability of B. Note that P(A∩B)=P(B∩A).

In the given problem, we have the following:

`\begin{gathered} P(A)=0.16 \\ P(B)=0.12 \\ P(A\cap B)=P(B\cap A)=0.11 \end{gathered}`

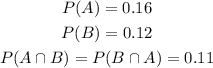
where A stands for athlete and B stands for biology major.

Thus, for P( biology major | athlete), we have the following:

`\begin{gathered} P(B|A)=(P(B\cap A))/(P(A)) \\ =(0.11)/(0.16) \\ =0.6875 \\ \approx0.688 \end{gathered}`

For P(athlete | biology major), we have the following:

`\begin{gathered} P(A|B)=(P(A\cap B))/(P(B)) \\ =(0.11)/(0.12) \\ =0.916\bar{6} \\ \approx0.917 \end{gathered}`