Question

The random variable x is normally distributed with a mean of 42 and a standarddeviation of 6.What is the approximate interquartile range for this variable?

Answer 1

Interquartile range is equal to the difference between the 75th percentile and 25th percentile, including in its interval 50% of the data.

If x is normally distributed, we have to calculate the z-value that includes 25% of the data, so that 50% of the data is within -z and z (in standard form).

The value of z that includes 50% of the data is z=+/- 0.6745

Then, we can calculate the limits of x as:

`\begin{gathered} x_1=\mu-z\cdot\sigma=42-0.6745\cdot6=42-4.05=37.95 \\ x_2=\mu+z\cdot\sigma=42+0.6745\cdot6=42+4.05=46.05 \end{gathered}`

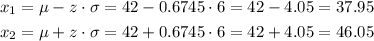
The interquartile range is [37.95, 46.05].