Question

Write the equation of the line, in slope-intercept form, through the following two points: (-11, -12) (-8, -21) slope: y-intercept: equation:

Answer 1

Given the points (-11, -12) and (-8,-21), you can calculate the slope of the line that passes through them using the following formula:

`m=(y_1-y_2)/(x_1-x_2)`
`m=(-12-(-21))/(-8-(-11))=(-12+21)/(-8+11)=(9)/(3)=3`

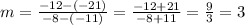
The slope is m=3

Now with one of the points and the slope you can determine the equation using the point slope form:

`y-y_1=m(x-x_1)`

(-11,-12)

`\begin{gathered} y-(-12)=3(x-(-11)) \\ y+12=3x+33 \\ y=3x+33-12 \\ y=3x+21 \end{gathered}`

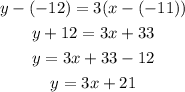
The y-intercept is the value of y when x=0, from the equation:

`\begin{gathered} y=3\cdot0+21 \\ y=21 \end{gathered}`

So,

The slope is 3

The y-intercept is 21

The equation is y=3x+21