Question

Find the scalar and vector projections of b onto a.a =(−3, 4)b =(7, 9)

Answer 1

Take into account that the scalar projection is given by:

`comp_a\vec{b}=\frac{\vec{a}\cdot\vec{b}}{a}`

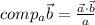
the magnitude of a vector is:

`\begin{gathered} a=\sqrt[]{(-3^{})^2+4^2} \\ a=\sqrt[]{25}=5 \end{gathered}`

The dot product between a and b is:

`\vec{a}\cdot\vec{b}=(-3,4)\cdot(7,9)=-3\cdot7+4\cdot9=-21+36=15`

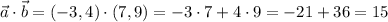
Then, the scalar projection is:

`\text{comp}_a\vec{b}=(15)/(5)=3`

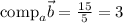
Now, consider that the vector projections is given by:

`\begin{gathered} \text{proj}_a\vec{b}=(\frac{\vec{a}\cdot\vec{b}}{a})(a)/(\lvert a\rvert) \\ \text{proj}_a\vec{b}=(3)/(5)\cdot(-3,4)=(-(9)/(5),(12)/(5)) \end{gathered}`

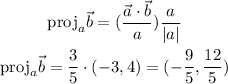
Hence, the answer is:

scalar projection of b onto a = 3

vector projection of b onto a = (-9/5 . 12/5)