Question

How can I solve -6y+11x=-36 and -4y+7x=-24 with Elimination

Answer 1

`\begin{cases}11x-6y=-36\Rightarrow\text{ Equation 1} \\ 7x-4y=-24\Rightarrow\text{ Equation 2}\end{cases}`

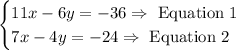
We can do the following steps to solve the system of equations using the elimination method.

Step 1: We multiply Equation 1 by 7 and the Equation 2 by -11.

`\begin{gathered} 11x-6y=-36\Rightarrow\text{ Equation 1} \\ (11x-6y)7=-36\cdot7 \\ \text{ Apply the distributive property} \\ 11x\cdot7-6y\cdot7=-252 \\ 77x-42y=-252 \end{gathered}`
`\begin{gathered} 7x-4y=-24\Rightarrow\text{ Equation 2} \\ (7x-4y)-11=-24\cdot-11 \\ 7x\cdot-11-4y\cdot-11=264 \\ -77x+44y=264 \end{gathered}`

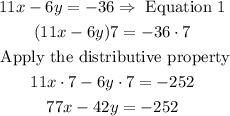
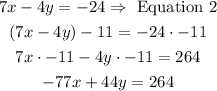
Step 2: We add the resulting equations.

`\begin{gathered} 77x-42y=-252 \\ -77x+44y=264\text{ +} \\ ------------- \\ 0x+2y=12 \\ 2y=12 \end{gathered}`

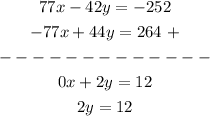
Step 3: We solve the above equation.

`\begin{gathered} \text{ Divide by 2 from both sides of the equation} \\ (2y)/(2)=(12)/(2) \\ $$\boldsymbol{y=6}$$ \end{gathered}`

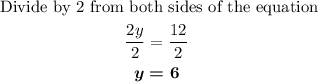
Step 4: We replace the obtained value in any initial equation. For example, in Equation 1.

`\begin{gathered} 11x-6y=-36\Rightarrow\text{ Equation 1} \\ 11x-6(6)=-36 \\ 11x-36=-36 \\ \text{ Add 36 from both sides} \\ 11x-36+36=-36+36 \\ 11x=0 \\ \text{ Divide by 11 from both sides} \\ (11x)/(11)=(0)/(11) \\ $$\boldsymbol{x=0}$$ \end{gathered}`

Therefore, the solution of the system of equations is the ordered pair (0,6).