Question

Assume that females have pulse rates that are normally distributed with a mean of 76.0 beats per minute and a standard deviation of 12.5 beats per minute.A) If 1 adult female is randomly selected, find the probability that her pulse rate is less than 83 beats per minute.

Answer 1

The problem is under normal distribution.

We need first to find the z-score in order to use the standard normal distribution table.

The formula of z-score is :

From the problem,

mean = 76

standard deviation = 12.5

and we are looking for x < 83 (Take note of the word "less than")

Using the formula above :

`z=(83-76)/(12.5)=0.56`

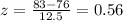
The z-score is 0.56

The normal distribution curve is :

So we will locate positive 0.56 and it is to the right of the centerline, if the z-score is negative we will use the other curve which is in negative side or left of the center line.

Using the table above, we will locate 0.56

0.56 = 0.5 + 0.06

First is to find 0.5 on the left side, and 0.06 on the top side.

The intersection is the probability which is also the area of the shaded region in the curve.

The intersection is 0.7123

Therefore, the probability is 0.7123