Question

A triangle has side of length a = 20 inches, b = 30 inches and c = 30 inches.Find angles A, B, C. Show triangle. Is this SAS or SSS?

Answer 1

Using cosine rule

`\begin{gathered} \cos A=(b^2+c^2-a^2)/(2* b* c) \\ \text{ =}(30^2+30^2-20^2)/(2*30*30)\text{ = }\frac{900\text{ + 900 - 400}}{1800}=(1400)/(1800) \\ \text{ = 0.7777} \\ A\text{ = }\cos ^(-1)0.7777=38.9^0\approx40^0 \end{gathered}`

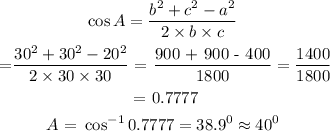
Since two sides of the triangles are equal, then the triangle is an issoseles triangle

In an issoseles triangle, two base angles are equal

This implies, angle at B = angle at C = x

Sum of angles in a triangle = 180 degrees

`\begin{gathered} \text{then } \\ x+x+40^0=180^0 \\ 2x=180^0-40^0 \\ 2x=140^0 \\ x\text{ =}(140^0)/(2)=70^0 \end{gathered}`

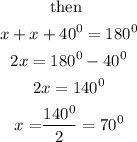
angle A = 40 degrees , angle B = 70 degrees , angle C = 70 degrees

The triangle is SAS , two sides are equal with an included angle

SSS is for triangles with equal sides