Question

a spring is stretched 2 cm when a mass of 40 grams is hung from it what is the spring constant of the spring

Answer 1

F = ma = -kx
m = 0.04 kg
x = -0.02m
a = 9.81m/s²

k = ma/x = 19.6 N/m

Answer 2

20kg/
`s^(2)` or 20N/m

Step-by-step explanation:

Applying Hooke's law which states that the force (F) needed to compress or extend a spring is directly proportional to the length of the extension or compression (x) i.e

=> F
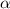`\alpha` x

=> F = k x ---------------------- (i)

Where k is the constant of proportionality called spring constant.

And from the question, the force is equal to the weight (W) of the mass (object).

=> F = W ----------------------- (ii)

where W = mg (mass x gravity)

Substituting W = mg into equation (ii) above, we have

F = mg

Substituting F = mg into equation (i) above, we have

mg = kx

Making k the subject of the formula, we have

k =
`(mg)/(x)` -------------------------------(iii)

Remember:

x is the extension = 2cm = 0.02m

m is the mass = 40g = 0.04kg

g is the acceleration due to gravity which we assume is 10m/
`s^(2)`

Substituting these values into equation (iii) above, we have

k =
`(0.04 x 10)/(0.02)`

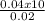
k = 20kg/
`s^(2)`

Therefore the spring constant is k = 20kg/
`s^(2)` or 20N/m

Note: kg/
`s^(2)` and N/m are both valid units for spring constant.