Question

Find the give term in the expanded form of the binomial?.

Answer 1

According to the binomial theorem, the k-th term of the binomial expansion of (a+b)^n is:

`\begin{pmatrix}n \\ k\end{pmatrix}a^(n-k)b^k`

Where k is an index that starts at 0 and ends at n.

To find the term number2 of (3y+1)^5, replace a=3y, b=1,n=5 and k=2:

`\begin{gathered} \begin{pmatrix}5 \\ 2\end{pmatrix}(3y)^(5-2)(1)^2=10\cdot(3y)^3(1) \\ =10\cdot3^3y^3 \\ =30y^3 \end{gathered}`

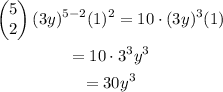
Therefore, the term 2 of the expanded form of the binomial (3y+1)^5 is 30y^3.