Answer:
• Account A has a principal of $100
,
• Account B has a principal of $700
,
• Account B earned you the most interest in the first month.
Step-by-step explanation:
Account A
• Simple Interest = $0.95
,
• Time = 3 months = 3/12 years
,
• Interest Rate=3.8%
We know that:

Account B
• Simple Interest = $21
,
• Time = 15 months = 15/12 years
,
• Interest Rate=2.4%
We know that:
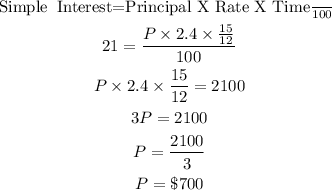
Next, we determine the account that earned you the most interest in the first month.
Account A (Interest in the First Month)
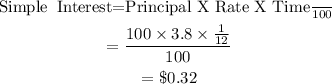
Account B (Interest in the First Month)
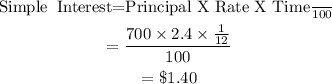
We see that Account B earned you the most interest in the first month.