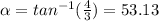Question

A vector has an x-component of length 3 and a y-component of length 4. What is the angle of the vector? (Hint: Use the inverse tangent.) A. 53.1 B. 48.6 C. 36.9 D. 76.0

Answer 1

A: 53.1

Inverse tan of the opposite side (y=4) divided by the adjacent side (x=3) gives:
The angle of the resulting vector is 53.1 degrees subtended from the x axis.

Answer 2

A. 53.1

Step-by-step explanation:

I incorporated a drawing of the exercise. In any right triangle we can use three trigonometric functions in order to find the angles that are different to 90 degrees.

This trigonometric functions are :

Sine (sin)

Cosine (cos)

Tangent (tan)

The hint to solve this exercise is to use the inverse tangent. The tangent is define as

`tan(\alpha)=(opposite)/(adjacent)`

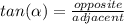
In the drawing the opposite side length is 4 and the adjacent side length is 3

⇒

`tan(\alpha)=(4)/(3)` ⇒

`\alpha=tan^(-1)((4)/(3))=53.13`