Question

9 and 10. find the slope of KM and ST. Determine if they are parallel, perpendicular,or neither .

Answer 1

9 is parallel and 10 is perpendicular

Explanation:

You find the slope by the change in y over the change in x

Points are in the form(x,y) You take the y numbers on top of the fraction and subtract them and you take the x numbers on the bottom of the fraction and subtract those. Simplify and you have the slope.

(-4,10) (2, -8)

`(-8 - 10)/(2 - - 4)` =
`(-18)/(2+4)` =
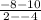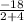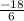`(-18)/(6)` Divide the top and bottom by 6

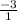`(-3)/(1)` = -3

The slope is -3. Let's compare to the other 2 points.

(1,2) (4, -7)

`(-7 - 2)/(4 - 1)` =
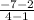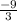`(-9)/(3)` = -3

The slopes are the same. When the slopes are the same, the lines are parallel.

10)

(-3, -7) (3,-3)

`(-3 - -7)/(3 - -3)` =
`(-3 + 7)/(3 + 3)` =
`(4)/(6)` =
`(2)/(3)`

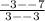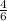(0,4) (6,-5)

`(-5 -4)/(6 - 0)` =
`(-9)/(6)` =
`(-3)/(2)`

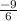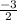
The slope are opposite reciprocals of each other so they are perpendicular.