Question

A shoreline observation post is located on a cliff such that the observer is 300 feet above sea level. The observer spots a ship approaching the shore and the ship is traveling at a constant speed.To input your angle measures in degrees, use the functions sind (5), cosd (5) and tand (5), instead of sin (5), cos (5) and tan (5).When the observer initially spots the ship, the angle of depression for the observer's vision is 5 degrees. At this point in time, how far is the ship from the shore? _____feet   After watching the ship for 46 seconds, the angle of depression for the observer's vision is 12 degrees. At this point in time, how far is the ship from the shore?____ feet   What is the ship's constant speed (in ft/sec)?____ ft/sec   If the ship continues traveling at this constant speed, how many seconds after the observer initially spotted the ship will the ship crash into the shore?____ sec

Answer 1

Given, the angle of depression for the observer's vision, α=5 degrees.

The height of the observer above sea level, h=300 feet.

Let x be the distance of the ship from the shore.

Using trigonometric property,

`\begin{gathered} \tan \alpha=\frac{opposite\text{ side}}{adjacent\text{ side}} \\ \tan \alpha=(h)/(x) \\ x=(h)/(\tan \alpha) \\ =(300)/(\tan 5^(\circ)) \\ =3429\text{ f}eet \end{gathered}`

The ship is 3429 feet from the shore when the angle of depression for the observer's vision is 5 degrees.

After watching the ship for 46 seconds, the angle of depression for the observer's vision, α=12 degrees. Let x be the distance of the ship form the shore when the angle of depression for the observer's vision is 12 degrees. Using trigonometric property,

`\begin{gathered} \tan \alpha=(h)/(x) \\ x=(h)/(\tan \alpha) \\ =(300)/(\tan 12^(\circ)) \\ =1411.39\text{ f}eet \end{gathered}`

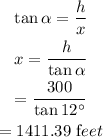
Therefore, the ship is 1411.39 feet from the shore when the angle of depression for the observer's vision is 12 degrees.

The distance travelled by the ship in t=46 s is

`\begin{gathered} d=3429\text{ -1411.39 } \\ =2017.61\text{ ft} \end{gathered}`

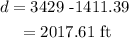
Hence, the speed of the ship is,

`\begin{gathered} s=(d)/(t) \\ =\frac{2017.61\text{ ft}}{46\text{ s}} \\ =43.86\text{ ft/s} \end{gathered}`

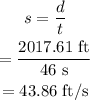
The speed of the ship is obtained as 43.86 ft/s.

When the observer initially spotted the ship, the distance from the shore to the ship is x=3429 ft.

The time taken by the ship to crash into the shore after the observer initially spotted the ship is,

`\begin{gathered} t=(x)/(s) \\ =\frac{3429\text{ f}t}{43.86\text{ ft/s}} \\ =78.18\text{ s} \end{gathered}`

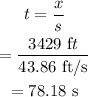
Therefore, the ship crash ito the shore after 78.18 s from the time the observer initially spotted the ship.