Question

Jeff has a balance of $2,513.77 on his credit card. He would like to pay off his card over the course of a year and a half by making identical monthly payments. The APR on his card is 10.66%, compounded monthly. Assuming that Jeff makes no additional purchases with his card, how much will he have to pay every month to reach his goal? (Round all dollar values to the nearest cent.) a. $151.73 b. $162.57 c. $139.65 d. $147.64

Answer 1

2513.77=X[(((1-(1+0.1066/12)^(-12*1.5))/(0.1066/12)]
Solve for x
X=151.73

Answer 2

a. $151.73

Explanation:

This question is based on EMI

The EMI formula is :

`(p*r*(1+r)^(n) )/((1+r)^(n)-1 )`

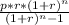
Here , p = 2513.77

n = 18 (year and a half)

r =
`(10.66)/(12)=0.888` ;

=>
`(0.888)/(100)=0.0088`

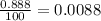
Putting the values in formula:

`(2513.77*0.0088*(1.0088)^(18) )/((1.0088)^(18)-1 )`

=$151.63 ; this amount is closest to $151.73

Therefore, Jeff will have to pay $151.73 every month to reach his goal.