Question

For the function, find the intervals where it is increasing and decreasing, and JUSTIFY your conclusion. Construct a sign chart to help you organize the information, but do not use a calculator. f(x) = xex 1

Answer 1

First we have to derivate the function

`\begin{gathered} f(x)=xe^{(1)/(x)}\rightarrow \\ f^(\prime)(x)=e^{(1)/(x)}+x\cdot(e^{(1)/(x)}\cdot(-1)/(x^2))=e^{(1)/(x)}(1-(1)/(x)) \end{gathered}`

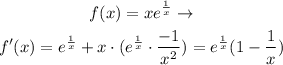
We have to find when the derivate is 0

`e^{(1)/(x)}(1-(1)/(x))=0\rightarrow1-(1)/(x)=0\rightarrow x=1`

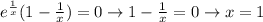
we have to consider x=0, because we can not divide by 0

so for a number lower than 0 we get that

`f^(\prime)(x)>0`

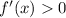
so it is increasing

for a number between 0 and 1 we get

`f^(\prime)(x)<0`

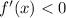
so it is decreasing

for a number greater than 1 we get

`f^(\prime)(x)>0`

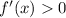
it is increasing