Question

Please do this step-by-step graph it by the table or at least explain how to do it

Answer 1

We are given a table of velocity vs time. We are asked the following:

Part a. We are asked to determine the average acceleration. To do that we will use the following formula:

`a=(\Delta v)/(\Delta t)=(v_f-v_0)/(t_f-t_0)`

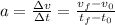
Where:

`\begin{gathered} a=\text{ acceleration} \\ v_f,v_0=\text{ final and initial velocities} \\ t_f,t_0=\text{ final and initial time} \end{gathered}`

Now, we use the final and initial values of velocity and time from the table.:

`a=(1.14(mm)/(ms)-0.2(mm)/(ms))/(650ms-50ms)`

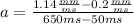
Solving the operations:

`a=0.0016(mm)/(ms^2)`

Therefore, the acceleration is 0.0016 mm/ms^2.

To convert to m/s^2 we will use the following conversion factors:

`\begin{gathered} 1000mm=1m \\ 1000ms=1s \end{gathered}`

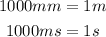
Now, we multiply by the conversion factors:

`a=0.0016(mm)/(ms^2)*(1m)/(1000mm)*((1000ms)/(1s))^2`

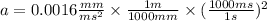
Solving the operations;

`a=1.6(m)/(s^2)^{}`

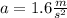
Part B. We are asked to determine the displacement. The displacement in a graph of velocity vs time is equivalent to the area under the curve of the graph, like this:

The area under the curve is approximately the area of a triangle, therefore, its area is given by:

`d=(bh)/(2)`

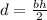
Where the base is the difference in time and the height is the difference is velocity, therefore, we have:

`d=(\Delta t\Delta v)/(2)=((t_f-t_0)(v_f-v_0))/(2)`

Substituting the values we get

`d=((650ms-50ms)(1.14(mm)/(ms)-0.2(mm)/(ms)))/(2)`

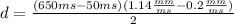
Solving the operations:

`d=282mm`

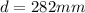
Now, we convert mm to cm using the following conversion factor:

`10mm=1cm`

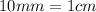
Multiplying by the conversion factor we get:

`d=282mm*(1cm)/(10mm)=28.2cm`

Therefore, the displacement is 28.2 cm.

Part C. This is a uniformly accelerated motion or a motion with a constant acceleration. We can tell from the graph because in this type of motion the graph of velocity vs time is a straight line. The slope of the line is the acceleration of the motion due to the fact that the acceleration does not change with time.