Question

Find the limit. Use l'Hospital's Rule if appropriate. If there is a more elementary method, consider using it.

lim (2x + 1)^(cot(x))
x→0+

Answer 1

`(2x+1)^(\cot x)=\exp\left(\ln(2x+1)^(\cot x)\right)=\exp\left(\cot x\ln(2x+1)\right)=\exp\left((\ln(2x+1))/(\tan x)\right)`

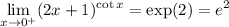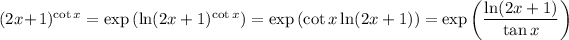
where
`\exp(x)\equiv e^x`.

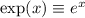
By continuity of
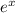
`e^x`, you have


`\displaystyle\lim_(x\to0^+)\exp\left((\ln(2x+1))/(\tan x)\right)=\exp\left(\lim_(x\to0^+)(\ln(2x+1))/(\tan x)\right)`

As
`x\to0^+` in the numerator, you approach
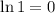
`\ln1=0`; in the denominator, you approach
`\tan0=0`. So you have an indeterminate form
`\frac00`. Provided the limit indeed exists, L'Hopital's rule can be used.


`\displaystyle\exp\left(\lim_(x\to0^+)(\ln(2x+1))/(\tan x)\right)=\exp\left(\lim_(x\to0^+)\frac{\frac2{2x+1}}{\sec^2x}\right)`

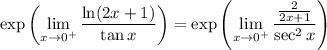
Now the numerator approaches
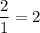
`\frac21=2`, while the denominator approaches
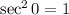
`\sec^20=1`, suggesting the limit above is 2. This means


`\displaystyle\lim_(x\to0^+)(2x+1)^(\cot x)=\exp(2)=e^2`