Question

Hello I am not able to solve this answer to determine the sequences or diverges to this question

Answer 1

We are given the following sequence:

`a_n=\mleft\lbrace(3)/(4),(1)/(8),(-1)/(2),(-9)/(8)\mright\rbrace`

We notice that each term is determined by adding -5/8 to the previous term, like this:

`\begin{gathered} (3)/(4)-(5)/(8)=(1)/(8) \\ \\ (1)/(8)-(5)/(8)=-(1)/(2) \\ \\ -(1)/(2)-(5)/(8)=-(9)/(8) \end{gathered}`

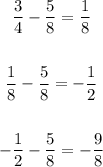
Therefore, the sequence is an arithmetic sequence with a common difference of -5/8.

Arithmetic sequences are convergent only when the common diference is zero, therfore, the sequence is divergent.