Step 1:
Line A and line B are parallel if the slope of line A is equal to the slope of line B
Line A and line B are perpendicular if the product of the slope of line A and the slope of line B is equal to - 1.
Step 2:
Line A has points (-1,3) and (5,1)
Line B has points (-2,3) and (0,9)
Next, find the slope of lines A and B

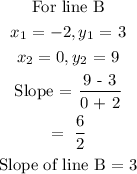
Step 3:
Line A is not parallel to line B since the slope of A is not equal to the slope of B.
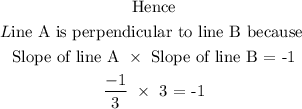
Final answer
The lines are perpendicular.