Question

Use this tool to make a scatter plot for the data provided and to create linear regression equation. 0246810-22-19-15-11-6-2Type your linear regression line of best fit in slope intercept form (y=mx+b). Do not put any spaces between your characters and round your values to the nearest hundredth y=Answer.Does the data appear to be linear? AnswerUse this tool to find the r-value. Type the r value to the nearest thousandth

Answer 1

Let us start by plotting the graph of years (x-values) and imports (y-values).

From the graph above, we can conclude that the trend appears linear. I started the year by replacing 1992 by 2yrs, 1994 by 4yrs, etc.

Therefore, the formula for the linear regression line is,

`y=ax+b`

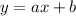
where,

`\begin{gathered} a=409.503\approx409.50(nearest\text{ hundredth)} \\ b=1109.82_{_{_{_{_{_{}}}}}} \end{gathered}`

Therefore, the linear regression line is

`y=409.50x+1109.82`

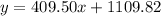
Now let us get the year import will exceed 12,000 by substituting the values of y to be 12,000 in the equation above.

`\begin{gathered} _{_{_{_{}}}}12000=409.50x+1109.82 \\ 12000-1109.82=409.50x \\ 10890.18=409.50x \\ (10890.18)/(409.50)=(409.50x)/(409.50) \\ 26.594=x \\ \Rightarrow x=26.594\approx27(nearest\text{ whole number)} \end{gathered}`

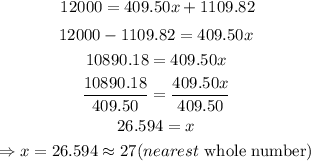
Hence, the year in which the import will exceed 12,000 will be in the year 2017.

Finally, from the graph we find the value of r to be 0.9848.