Question

Which tables have a lower unit rate than the rate represented in the equation? y=3/5xThe pictures are the answer choices.

Answer 1

The unit rate represented in the equation y = 3/5x is the value multiplying x, that is, the unit rate is 3/5.

In order to find the unit rate of each table, we just need to divide the y-values by the x-values.

So, the unit rate of the first table is:

`(y)/(x)=(4)/(3)`

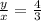
The value 4/3 is greater than the value 3/5.

In the second table we have that:

`(y)/(x)=(4)/(5)`

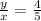
The value 4/5 is greater than the value 3/5;

In the third table, we have that:

`(y)/(x)=(18)/(31)`

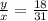
In order to compare this fraction with 3/5, we need to make them be in the same denominator, so we have to calculate the least common multiple of the denominators.

Since 5 and 31 are prime numbers, the LCM is the product of both numbers.

So we have that:

`\begin{gathered} (18)/(31)=(18\cdot5)/(31\cdot5)=(90)/(155) \\ \\ (3)/(5)=(3\cdot31)/(5\cdot31)=(93)/(155) \end{gathered}`

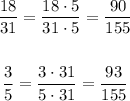
The number 90/155 is lesser than 93/155, therefore 18/31 is lesser than 3/5, so the answer is the third table.