Question

Determine which is the better investment: 5.02% compounded semiannually or 4.95% compounded quarterly. Round youranswers to 2 decimal places.The 5.02% semiannual investment gives an effective rate of %.The 4.95% quarterly investment gives an effective rate of %.One more part which investment is the better one?

Answer 1

Step 1:

In this question, we have the following:

Step 2:

Using the sample solution in the second picture, we can see that the

The Formula of the Effective Interest Rate is given by:

`\begin{gathered} \text{E = ( 1+}(r)/(n))^{n\text{ }}-1 \\ \text{where r = Rate } \\ In\text{ this case, we have that r = 5.02\% = 0.0502 } \\ n\text{ =2 , showing the semi-annual }compounded\text{ rate} \end{gathered}`

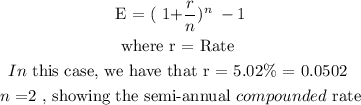
In this case, the Effective Interest Rate, E = 0.05083001 %

`E\text{ }\approx\text{ 0.05 \% ( to two decimal places)}`

Step 3:

`\begin{gathered} \text{ E= ( 1+}(r)/(n))^{n\text{ }}-1 \\ \text{where E=Effective rate} \\ n\text{ = number of periods per year the interest is calcuated = 4} \\ r=\text{ Interest rate per year = 4}.95\text{ \%} \end{gathered}`

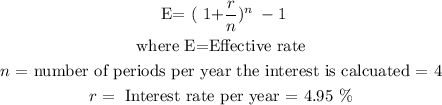
Then, the detailed solution is as follows:

In this case, the Effective Interest Rate, E = 0. 05042

`E\text{ }\approx0.05\text{ \% (to 2 decimal places)}`

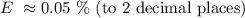
Step 4:

One more part which investment is the better one?

Despite the two values are:

`E\approx0.05\text{ \% (to 2 decimal places)}`

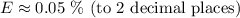
But for the semi-annual investment, E= 0.05083001 %

and the quarterly investment, E = 0.05042%

CONCLUSION:

The better investment is that of the semi-annual investment because it is bigger than the quarterly investment.