We have here a right triangle. We can use trigonometric ratios (sine, cosine, or tangent) to find the length of any side of the triangle.
To do this, we can use angle 77 as a reference angle to find the side JK (the value of x, in this case.)
It is also important the largest side of a right triangle is the hypotenuse (JK).
Having this information, we can proceed as follows:
LJ = 6.9 feet
x = ?
We know that the opposite side to the angle 77 is the side LJ, and knowing this, we can use the sine ratio:
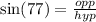
Sine is the opposite side over the hypotenuse, then we have:
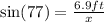
Solving for x, we need to multiply the equation by x to both sides of the equation:
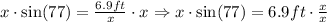
Since x/x = 1. We can now divide both sides of the equation by sin(77):
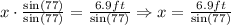
And the value for x (the side JK) is:

Rounding to the nearest tenth, we have that the value for x, the length of JK = 7.1 feet.