A quadratic function in standard fomr is:
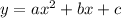
a is the number multiplying the quadratic term. b is the number multiplying the x and c is the term without x.
In this case we have:
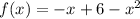
Now if we rearrange as above:
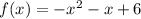
And now we can clearly see that:
a = -1
b = -1
c = 6
now for the table we need to input each term in it. The third row in the x section of the table will be always 6, because is a constant which does not depend of x.
We need to use additional values to the given ones to complete the interval [-4, 3].
We'll use:
-4, -3, -2, -1, -0.5, 0, 1, 2
Now, for -x², the row is:
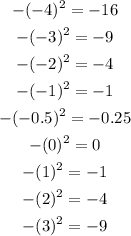
The -x row is:
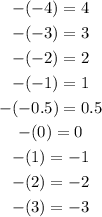
Finally we need to complete the y row. This is the sum of the three values in the x:
The y row is:
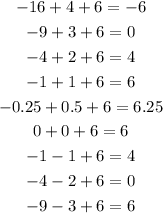
With that, the table is complete, and the problem is solved.