From the problem, we have a line segment that is 7 inches long.
It is divided into two segments (long and short) in which the ratio of long segment to the short segment is the golden ratio, Ф = 1.618
Let x = shorter segment
and the longer segment will be (7 - x)
The ratio will be :

Solve for x :
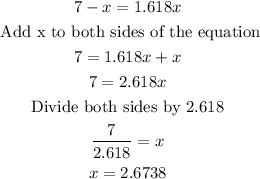
Substitute x = 2.6738 to the equation of long segment :
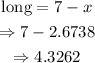
The answer rounded to 2 decimal places is 4.33 inches