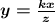Direct variation is a relationship between two variables. In this case, y is directly proportional to x. We say y varies directly with x if:
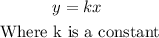
On the other hand, inverse variation is another relationship between two variables. In this case, y is inversely proportional to x. We say y varies inversely with x if:
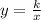
Now, if we combine the two previous definitions, we have:
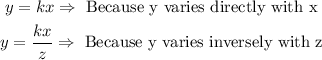
Therefore, y varies directly as x and inversely as z can be modeled by the equation