Question

Marie has two options to buy the new carpet, but in both of them she has leftover material and she has to cut a piece of it. The first option she has is to buy a roll that is 10 feet wide and 10 feet long, at a cost of $3.50 per square foot. And the second option is to buy a roll that is 9 feet wide and 11 feet long, at $3.25 per square foot. Which would be the best option?

Answer 1

The second option is the best option

Step-by-step explanation:

Given the dimensions of the roll in the first option;

Length(l) = 10 feet

Width(w) = 10 feet

So the area(A) of the roll is;

`A=l*w=10*10=100\text{ ft}^2`

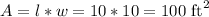
Given the cost per square foot as $3.50, therefore the cost for 100 square feet roll will be $350

Given the dimensions of the roll in the second option as;

Length (l) = 11 feet

Width (w) = 9 feet

So the area(A) of the roll is;

`A=l*w=11*9=99\text{ ft}^2`

Given the cost per square foot as $3.25, therefore the cost for 99 square feet roll will be $321.75

We can see that the second option is cheaper since she would have to spend $321.75 for the roll as against a roll that cost $350 for the first option.

So the second option is the best option.