Question

Create a transformation that is not a similarity transformation. Explain why your transformation wouldn’t be similar

Answer 1

Two figures are similar if they have the same shape. This means that two similar figures have the same internal angles although their size and orientation may be different. A similarity transformation maps a figure onto another figure that is similar. Here we must find a non-similarity transformation i.e. a transformation that maps a figure onto a non-similar figure. In order to see this let's build any figure in the coordinate plane, for example, a triangle:

Now let's define a transformation, i.e. an operation over the coordinates of the points in the grid. Let's use (x,y) for any generic point and the transformation is:

`(x,y)\rightarrow(x,(y)/(2))`

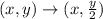
So this tranformation takes a point and divides its y-coordinate by 2. Let's apply it to the three points that define the triangle in the figure:

`\begin{gathered} A=(0,0)\rightarrow A^(\prime)=(0,(0)/(2))=(0,0) \\ B=(3,0)\rightarrow B^(\prime)=(3,(0)/(2))=(3,0) \\ C=(3,3)\rightarrow C^(\prime)=(3,(3)/(2))=(3,1.5) \end{gathered}`

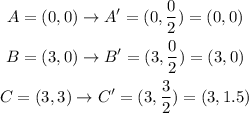
Then we graph the new transformed triangle A'B'C':

As you can see two of the angles of A'B'C' don't match with the angles of ABC. This means that ABC and A'B'C' are not similar. Therefore this transformation is not a similarity transformation.

In summary, the answer is that the transformation (x,y)⇒(x,y/2) is not a similarity transformation because it doesn't keep the shape of the figures it transforms.