Question

8x – 12y = – 24 a. x-intercept: b. y-intercept: 10+ C. graph 9 8 7 6 5 4 13 2 -10 -9 -8 -7 -6 -5 -4 -B-2 -1 2 15 4 SI 6 8 9 10 -2 3 -6 -7 -8 9 10 Clear All Draw:

Answer 1

To determine the x- and y-intercepts is best to write the equation in slope-intercept form.

Given

`8x-12y=-24`

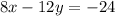
-Pass the x-term to the right side of the equation by applying the opposite operation to both sides of it:

`\begin{gathered} 8x-8x-12y=-8x-24 \\ -12y=-8x-24 \end{gathered}`

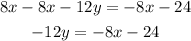
-Divide both sides of the equal sign by -12

`\begin{gathered} (-12y)/(-12)=(-8x)/(-12)-(24)/(-12) \\ y=(2)/(3)x+2 \end{gathered}`

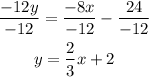
So the equation in slope-intercept form is:

`y=(2)/(3)x+2`

a) The x-intercept is the point where the line crosses the x-axis, at this point, the y-coordinate is equal to zero. To determine the x-coordinate of the intercept, you have to equal the equation to zero and calculate the corresponding value of x:

`0=(2)/(3)x+2`

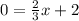
-Subtract 2 to both sides of the equal sign

`\begin{gathered} 0-2=(2)/(3)x+2-2 \\ -2=(2)/(3)x \end{gathered}`

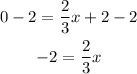
-Multiply both sides of the expression with the reciprocal fraction of 2/3

`\begin{gathered} (-2)(3)/(2)=((2)/(3)\cdot(3)/(2))x \\ -3=x \end{gathered}`

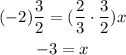
The x-intercept is (-3,0)

b) The y-intercept is the point where the line crosses the y-axis, at this point, the x-coordinate is equal to zero. To determine the y-intercept, replace the equation with x=0 and calculate the corresponding value of y:

`\begin{gathered} y=(2)/(3)x+2 \\ y=(2)/(3)\cdot0+2 \\ y=2 \end{gathered}`

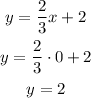
The y-intercept is (0,2)

c) To graph the line, plot both intercepts on the coordinate system and then link both points with a line: