Question

The speed of train A is 20 mph slower than the speed of train B. Train A travels 230 miles in the same time it takes train B to travel 330 miles. Find the speed of each train.

Answer 1

recall your d = r*t or distance = rate * time

so... let's say B is going at a speed of "r"
A is slower than B by 20mph, or whatever "r" is, A's speed is r - 20

at a certain time "t", A runs 230 miles whilst B runs 330 miles, same "t" time

so.. hmmm
`\bf \begin{array}{lccclll} &distance&rate&time\\ &-----&-----&-----\\ A&230&r-20&t\\ B&330&r&t \end{array}\\\\ -----------------------------\\\\ \begin{cases} 230=(r-20)(t)\\\\ 330=(r)(t)\implies (330)/(r)=\boxed{t}\\ ----------\\ 230=(r-20)\left( \boxed{(330)/(r)} \right) \end{cases}`

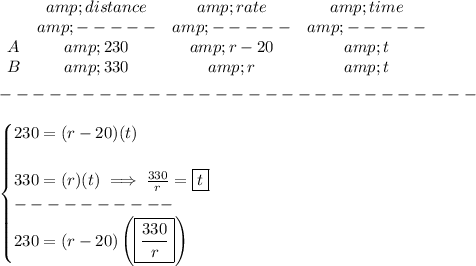
solve for "r", to see how fast B was going

what about A? well, A's speed is r - 20