Question

Find the expected value of the winningsfrom a game that has the followingpayout probability distribution:Payout ($) 1 2 5 8 10Probability 0.35 0.2 0.1 0.2 0.15Expected Value = [?]Round to the nearest hundredth.

Answer 1

On the frequency table, you can see the payouts of the lottery and the corresponding probabilities for every payout. To determine the expected payout, you have to multiply each possible price by its corresponding probability and add the results, following the formula:

`E(X)=\sum ^(10)_(n\mathop=1)x_i\cdot P(x_i)`
`\begin{gathered} E(X)=(1\cdot0.35)+(2\cdot0.20)+(5\cdot0.10)+(8\cdot0.20)+(10\cdot0.15) \\ E(X)=0.35+0.4+0.5+1.6+1.5 \\ E(X)=4.35 \end{gathered}`

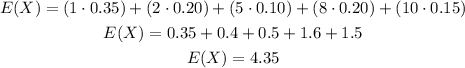
The expected payout for the lottery is $4.35