Question

A rectangle is 20 meters long and 20 meters wide. If the length is increased by 10% and the width is decreased by 10%, what is the new area?

Answer 1

Consider that the initial length and width of the rectangle are given as,

`\begin{gathered} l=20 \\ b=20 \end{gathered}`

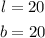
After the length is increased by 10%, the new length (L) of the rectangle is calculated as,

`\begin{gathered} L=(1+(10)/(100))\cdot l \\ L=((110)/(100))\cdot20 \\ L=22 \end{gathered}`

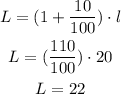
After the width is decreased by 10%, the new width (B) of the rectangle is calculated as,

`\begin{gathered} B=(1-(10)/(100))\cdot b \\ B=((90)/(100))\cdot20 \\ B=18 \end{gathered}`

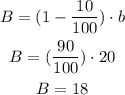
Then the area (A) of the new rectangle is calculated as,

`\begin{gathered} A=L* B \\ A=22*18 \\ A=396 \end{gathered}`

Thus, the new area of the rectangle is 396 square meters.