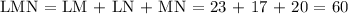Question

If L, M and N are the midpoints of the sides of the triangle PQR, PR= 46, PQ = 40, and LN = 17, find each measure.; LM, MN, QR and the perimeter of LMN

Answer 1

LM = 23; MN = 20 ; QR = 34 ; Perimeter of LMN is 60

Here, we want to find the measures

From what we have, triangle LMN is formed by joining the midpoints of the triangle PQR

From the midpoint theorem, the sides of PQR are parallel and exactly half the measure of the sides they face

For example, LM is half PR

a) LM

`LM\text{ = }(1)/(2)* PR\text{ = }(1)/(2)*46\text{ = 23}`

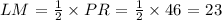
b) MN

`MN\text{ = }(1)/(2)* PQ\text{ = }(1)/(2)*\text{ 40 = 20}`

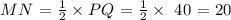
c) QR

Here, QR will be twice the measure of LN

`QR\text{ = 2}* LN\text{ = 2}*17\text{ = 34}`

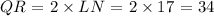
d) To find the perimeter of LMN, we have to add up the measure of the side lengths

We have this as;

`\text{LMN = LM + LN + MN = 23 + 17 + 20 = 60}`