Question

Solve: 9x^2+2x= -3 , using the quadratic formula

Answer 1

Given the question in the question tab, the following are the solution steps to answer the question.

STEP 1: Write the given quadratic equation.

`9x^2+2x=-3`

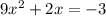
STEP 2: Express the equation in the standard quadratic form

`\begin{gathered} \text{standard form}=ax^2+bx+c=0 \\ 9x^2+2x=-3 \\ \text{Add 3 to both sides} \\ 9x^2+2x+3=-3+3 \\ 9x^2+2x+3=0 \end{gathered}`

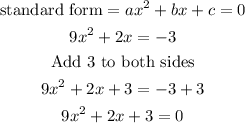
STEP 3: Write the quadratic formula

`x_1,x_2=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

STEP 4: Write the needed parameters to substitute into the formula

`\begin{gathered} 9x^2+2x+3=0 \\ a=9,b=2,c=3 \end{gathered}`

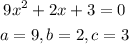
STEP 5: Substitute the values into the formula and solve for x

`\begin{gathered} x_(1,2)=(-2\pm√(2^2-4\cdot\:9\cdot\:3))/(2\cdot\:9) \\ \text{simplify }\sqrt[]{2^2-4\cdot\: 9\cdot\: 3} \\ \sqrt[]{2^2-4\cdot\: 9\cdot\: 3}=\sqrt[]{4-108}=\sqrt[]{-104}=2√(26)i \\ x_(1,\: 2)=\frac{-2\pm\sqrt[]{2^2-4\cdot9\cdot3}}{2\cdot\: 9}=(-2\pm\:2√(26)i)/(2\cdot\:9) \\ \mathrm{Separate\: the\: solutions} \\ x_1=(-2+2√(26)i)/(2\cdot\:9),\: x_2=(-2-2√(26)i)/(2\cdot\:9) \\ \frac{-2+2\sqrt[]{26}i}{2\cdot\: 9}=-(1)/(9)+\frac{\sqrt[]{26}}{9}i_{} \\ \frac{-2-2\sqrt[]{26}i}{2\cdot\: 9}=-(1)/(9)-\frac{\sqrt[]{26}}{9}i \\ x=-(1)/(9)+\frac{\sqrt[]{26}}{9}i\text{ or }-(1)/(9)-\frac{\sqrt[]{26}}{9}i \end{gathered}`

Hence, the roots of the equations are:

`\begin{gathered} x_1=-(1)/(9)+\frac{\sqrt[]{26}}{9}i \\ x_2=-(1)/(9)-\frac{\sqrt[]{26}}{9}i \end{gathered}`