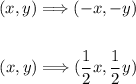Question

Which sequence of transformations shows that ABC is similar to A'B'C'?

Answer 1

From the figure, we have the coordinates of the vertices:

• ABC ==> A(0, 4), B(2, 0), C(0, 0)

• A'B'C' ==> A'(0, -2), B'(-1, 0), C'(0, 0)

Let's determine the sequence of transformations which shows that ABC is similar to A'B'C'.

The sequence of transformation which occured here is a rotation followed by a dilation.

For the rotation, apply the rules of rotation:

180 degrees rotation: (x, y) ==> (-x, -y)

We now have the coordinates:

(0, -4), (-2, 0), (0, 0)

Then for the dilation, let's find the scale factor.

To find the scale factor, divide the corresponding coordinates of A'B'C' by that of ABC:

`\begin{gathered} (A^(\prime))/(A^(\prime))=(0)/(0),(-2)/(-4)=(0,(1)/(2)) \\ \\ (B^(\prime))/(B)=((-1)/(-2),(0)/(0))=((1)/(2),0) \end{gathered}`

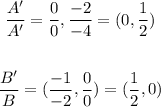
Therefore, the scale factor of the dilation is (1/2, 0)

Therefore, the sequence of transformations which shows that ABC is similar to A'B'C' are:

`\begin{gathered} (x,y)\Longrightarrow(-x,-y) \\ \\ (x,y)\Longrightarrow((1)/(2)x,(1)/(2)y) \end{gathered}`